1. Finding the Present value of a sum of money receivable in the future.
The present value (PV) of an investment refers to the current worth of a future sum of money, taking into consideration the time value of money. In other words, it’s the amount that needs to be invested today to achieve a specific future value, considering an assumed interest rate or discount rate. The formula for calculating the present value of an investment is:
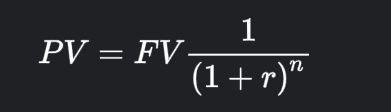
Where:
- PV = Present Value
- FV = Future Value (the amount you want to calculate the present value for)
- r = Interest Rate or Discount Rate per period (expressed as a decimal)
- n = Number of periods (usually years)
Here’s how you can calculate the present value of an investment step by step:
- Identify the Future Value (FV): Determine the future amount you expect to receive from the investment. This could be the maturity value of a bond, the final payout from a project, or any other expected future cash flow.
- Determine the Interest Rate (r): Determine the appropriate interest rate or discount rate to use. This rate reflects the opportunity cost of tying up funds in the investment or the cost of capital. Express this rate as a decimal.
- Define the Number of Periods (n): Determine the number of time periods for which the investment will accrue interest or discounting. This could be the number of years the investment will be held.
- Plug Values into the Formula: Insert the values of FV, r, and n into the present value formula. Make sure the interest rate and the number of periods are in consistent units (both annual, semi-annual, etc.).
- Calculate: Use a calculator or spreadsheet software to perform the calculations.
For example, let’s say you have an investment that will yield Rs. 1,00,000 in 5 years, and you want to calculate its present value at a 6% annual interest rate. Using the formula:
PV=100000 / (1+0.06)^5 = Rs. 74725.82
So, the present value of this investment is approximately Rs. 74725.82
Keep in mind that the present value calculation assumes that the future cash flows are known with certainty and that the interest rate remains constant over the investment period. If these assumptions don’t hold true, the actual present value could differ.
2. Finding the Present value of an ordinary annuity receivable in the future years.
The present value of an ordinary annuity is the current worth of a series of future cash flows, where each cash flow occurs at the end of each period. In other words, it’s the value of a stream of payments made at the end of each period over a specified time frame. To find the present value of an ordinary annuity, you can use the following formula:
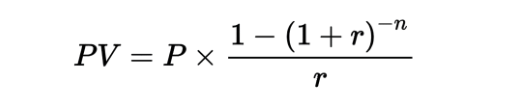
Where:
- PV is the present value of the annuity.
- P is the periodic payment amount (annuity payment) made at the end of each period.
- r is the periodic interest rate (expressed as a decimal).
- n is the total number of periods.
Here’s how you can use this formula step by step:
- Determine the values for P, r, and n.
- P: The amount of money you will receive or pay at the end of each period.
- r: The periodic interest rate. If you have an annual interest rate, you’ll need to divide it by the number of periods per year (e.g., if the annual interest rate is 6% and compounding is done monthly, r would be 0.005 i.e. 0.5%)
- n: The total number of periods the annuity will last.
- Plug the values into the formula.
- Calculate (1+r)^(−n). ( it can be done as[ 1 / (1+r)^n] )
- Subtract the result from step 3 from 1.
- Divide the result from step 4 by r.
- Multiply the result from step 5 by P.
The final result (PV) will be the present value of the ordinary annuity.
For example, let’s say you have an investment that will yield Rs. 1,00,000 each year for 5 years, and you want to calculate its present value at a 6% annual interest rate. Using the formula:
PV=100000 x [1- {(1+0.06)^-5}] / 0.06 = Rs. 421236.40
So, the present value of this ordinary annuity is approximately Rs. 421236.40
Remember that this formula assumes that payments are made at the end of each period. If payments are made at the beginning of each period, you would need to adjust the formula accordingly. Further please note that if annuity is increase at a certain percentage at each period then in above formula, in nominator “(1+r)^-n” is relaced by “(1+g)^n/(1+r)^n ” and in denominator r is replaced by (r-g) where g is the growth rate of annuity.
It’s worth noting that you can also use financial calculators, spreadsheet software (like Microsoft Excel or Google Sheets), or online present value calculators to simplify these calculations. These tools often have built-in functions that can directly compute the present value of an ordinary annuity.
3. Finding the Present value of an annuity due receivable in the future years.
The present value of an annuity due is the current worth of a series of future cash flows, where each cash flow occurs at the beginning of each period. In other words, it’s the value of a stream of payments made at the start of each period over a specified time frame. The formula to calculate the present value of an annuity due is:
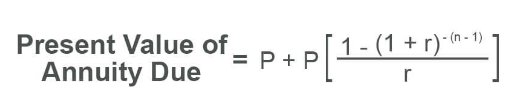
Where:
- PV is the present value of the annuity due.
- P is the periodic payment (annuity payment) made at the beginning of each period.
- r is the periodic interest rate (expressed as a decimal).
- n is the number of periods.
Here are the steps to calculate the present value of an annuity due:
- Identify the values: Determine the values for P (annuity payment), r (interest rate per period), and n (number of periods).
- Convert the interest rate: If the annual interest rate is given, make sure to convert it to the periodic interest rate by dividing it by the number of compounding periods per year.
- Plug the values into the formula: Substitute the values of P, r, and n into the formula for the present value of an annuity due.
- Perform calculations: Calculate the exponentials and perform the necessary calculations according to the formula.
- Calculate the present value: The result of the calculations will be the present value of the annuity due.
It’s important to note that the formula assumes a constant payment amount throughout the annuity’s duration and a constant interest rate. If either the payment amount or the interest rate changes over time, the calculation becomes more complex.
Let’s go through an example:
For example, let’s say you have an investment that will yield Rs. 1,00,000 each year for 5 years at the beginning of each period, and you want to calculate its present value at a 6% annual interest rate. Using the formula:
PV=100000+ [100000 x [1- {(1+0.06)^-4}] / 0.06] = Rs. 446510.60
So, the present value of this annuity due is approximately Rs. 446510.60
Remember that this formula assumes that the payments occur at the beginning of each period. If the payments were made at the end of each period, you would use a slightly different formula. Further please note that if annuity is increase at a certain percentage at each period then in above formula, in nominator “(1+r)^-(n-1)” is relaced by “(1+g)^(n-1)/(1+r)^(n-1) ” and in denominator r is replaced by (r-g) where g is the growth rate of annuity.