1. Finding the future value of a one-time investment.
The future value (FV) of an investment or cash flow represents the value of that investment or cash flow at a specified point in the future, taking into account the effects of interest or investment growth. The formula to calculate the future value is:
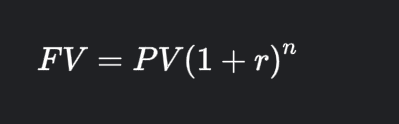
Where:
- FV = Future Value
- PV = Present Value or initial investment amount
- r = Interest rate or rate of return per period (expressed as a decimal)
- n = Number of periods
Here’s how you can calculate the future value:
- Identify the values:
- PV: The present value or initial investment amount.
- r: The interest rate or rate of return per period (expressed as a decimal).
- n: The number of periods.
- Plug in the values into the formula: FV=PV×(1+r)^n
- Calculate the future value.
Here’s an example:
Suppose you invest Rs. 1,00,000 at an annual interest rate of 6% for 5 years. You want to calculate the future value of this investment.
- PV = Rs. 1,00,000
- r=0.06 (6% as a decimal)
- n=5 years
Plug these values into the formula:
FV=100000×(1+0.06)^5 = 133822.6
Hence Rs. 133822.06 is the future value of investment of Rs. 100000 for 5 years at an interest rate of 6% p.a. with annual compounding.
Keep in mind that this formula assumes compounding of interest, which means that the interest earned in each period is added to the principal for the next period’s calculations. If interest is compounded more frequently (such as quarterly or monthly), adjustments to the formula are needed.
2. Future value of annuity/SIP made at the end of Each period i.e. Ordinary Annuity.
The future value of an annuity formula calculates the value of a series of equal payments made at regular intervals, compounded at a specific interest rate, over a defined period of time. The formula for calculating the future value of an annuity is as follows:
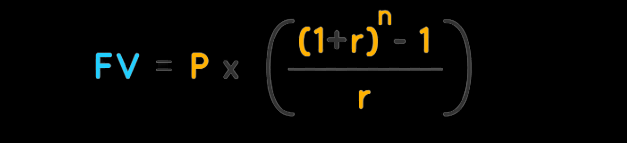
Where:
- FV is the future value of the annuity.
- P is the payment amount made at each compounding interval (annuity payment).
- r is the interest rate per compounding period (expressed as a decimal).
- n is the total number of compounding periods.
Let’s break down the components of the formula:
- Payment Amount (P): This is the amount of money you contribute to the annuity at each compounding interval. It remains constant throughout the annuity.
- Interest Rate per Compounding Period (r): The interest rate should be expressed as a decimal. For example, if the annual interest rate is 5%, then r would be 0.05
- Number of Compounding Periods (n): This represents the total number of compounding intervals or periods. It’s determined by the number of payments made over the life of the annuity.
The formula calculates the future value of the annuity by summing the future values of each individual payment, compounded at the given interest rate. The numerator {(1+r)^n}−1 represents the future value of an ordinary annuity factor. Dividing this by r then calculates the present value of an ordinary annuity factor. Multiplying this by the payment amount P gives the future value of the entire annuity.
Here’s an example:
Suppose you invest Rs. 1,00,000 every year at an annual interest rate of 6% for 5 years. You want to calculate the future value of this ordinary annuity.
- P = Rs. 1,00,000
- r=0.06 (6% as a decimal)
- n=5 years
Plug these values into the formula:
FV=100000× [{(1+0.06)^5}-1] / 0.06 = 563709.30
Hence Rs. 563709.30 is the future value of an ordinary annuity/SIP of Rs. 100000 for 5 years at an interest rate of 6% p.a. with annual compounding.
It’s important to note that this formula assumes regular, fixed payments and a consistent interest rate. Additionally, the formula assumes that payments are made at the end of each compounding period (an ordinary annuity). If payments are made at the beginning of each period (an annuity due), the formula would be slightly different.
Further please note that if there is any increase in annuity value in each period (knows as annuity growth rate) then in above formula number 1 is replaced by (1+g)^n and denominator is replace by (r-g).
This formula is useful for financial planning, investment analysis, and retirement planning, among other applications. If you’re working with a specific scenario, you can plug in the values for P, r, and n to calculate the future value of the annuity.
3. Future value of annuity/SIP made at the beginning of Each period i.e. Annuity due.
The formula for calculating the future value of an annuity made at the beginning of each period, also known as an “annuity due,” is a modification of the regular annuity formula. The main difference is in the timing of the payments. In an annuity due, payments are made at the beginning of each period rather than at the end. The formula for calculating the future value of an annuity due is as follows:
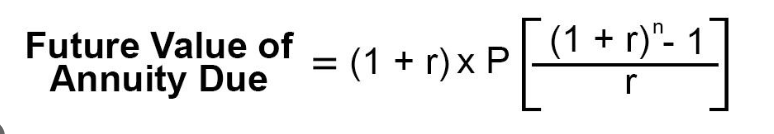
Where:
- FV is the future value of the annuity due.
- P is the payment amount made at the beginning of each compounding interval (annuity payment).
- r is the interest rate per compounding period (expressed as a decimal).
- n is the total number of compounding periods.
The formula is similar to the regular annuity formula, but there’s an additional multiplication factor of (1+r) in the numerator. This is because in an annuity due, each payment starts earning interest immediately, so the value of each payment at the end of the annuity period is (1+r) times greater than in a regular annuity.
Here’s how to use the formula step by step:
- Identify Values:
- Determine the payment amount P.
- Convert the annual interest rate to a decimal and use it for r.
- Determine the total number of compounding periods n.
- Calculate the Future Value of Ordinary Annuity: Use the regular annuity formula to calculate the future value of an ordinary annuity. This represents the value of the annuity if payments were made at the end of each period.
- Adjust for Annuity Due: Since payments are made at the beginning of each period in an annuity due, you need to multiply the future value of the ordinary annuity by (1+r) to account for the additional compounding period.
- Calculate the Future Value: Multiply the result from step 3 by the payment amount P to get the future value of the annuity due.
Remember that the interest rate r and the compounding period n should match in terms of time unit (e.g., both should be annual, semi-annual, etc.). Additionally, ensure that the payment amount P remains consistent with the time period used for r and n.
Here’s an example:
Suppose you invest Rs. 1,00,000 every year at an annual interest rate of 6% for 5 years. You want to calculate the future value of this annuity due.
- P = Rs. 1,00,000
- r=0.06 (6% as a decimal)
- n=5 years
Plug these values into the formula:
FV= (1+0.06) x 100000 × [[{(1+0.06)^5}-1] / 0.06] = 597531.90
Hence Rs. 597531.90 is the future value of an annuity due/SIP of Rs. 100000 for 5 years at an interest rate of 6% p.a. with annual compounding.
This formula is especially useful for scenarios where payments are made at the beginning of each period, such as some lease agreements, rental payments, and investments. Further please note that if there is any increase in annuity value in each period (knows as annuity growth rate) then in above formula number “-1” is replaced by “-(1+g)^n” and denominator is replace by (r-g).

